There are several ways to approximate the cost of a leaky house. Before I proceed, however, I just want to mention there are other much larger longterm monetary benefits to building a tight house, like a longer lifecycle and less maintenance due to keeping critters and moisture out of the building enclosure. I’m sure there’s a way to calculate the value of these additional benefits, but it’s beyond my abilities (and you’d have to use a lot of assumptions to get there).

Having gone through the numbers, there is some value to understanding the factors that are used to estimate the cost of infiltration, as well as the proportion of infiltration cost to the overall energy costs required to heat a house.

The primary method to estimate infiltration is to calculate the energy required to condition the air replaced by infiltrated air. First we must convert the ACH50 value derived from the blower door test to a ‘natural’ value (ACHnat) that might be experienced when the house is not being pressurized by artificial means. Then calculate the energy required to heat the naturally infiltrating air on a yearly basis. Then calculate the cost of the energy used.

Converting ACH50 to ACHnat is based on many assumptions including the climate zone, height of the house and the degree to which the house is shielded from the wind by surrounding vegetation and other structures. Energy Star recommended a value of 17.8 for ‘well shielded’, 14.8 for ‘normal’ and 13.3 for ‘exposed’ locations in Zone 2. Let’s use the normal value for now.

Dividing 1 ACH50 by 14.8 equals 0.067 ACHnat. This means approximately 6-7% of the air volume in the house (992 ft3) will be replaced by infiltration each hour. [This is not entirely accurate since the volume of the basement is included as conditioned space, yet it is not heated directly.] Let’s assume the inside temperature is 65°F and the outside temperature is 10°F. It takes 0.018 BTUs per hour to heat 1 cubic foot of air 1°F. 992 * 0.018 * 55°F = 982 BTU/hr to heat the infiltrated air.

1 BTU equals 0.293 Watts, so 992 * 0.293 = 288 Watts or 0.288 kW. At $0.14/kWh it would cost $0.04/hr while the temperature outside is 10°F. (We’ll take into account that an air source heat pump (ASHP) can be 2 to 3 times more efficient at converting a kW to a BTU later.)

We can use heating degree days (HDD) to estimate the cost over a typical heating season. In our climate area we have 7100 HDD in a heating season. Substituting 7100 for 55 in the above calculations and multiplying times 24 to get a daily value means you would spend roughly $125 for one heating season just to heat infiltrated air.

But as I mentioned earlier, an air-source heat pump can be 2 to 3 times more efficient at converting electricity to heat than simply using electrical resistance. There are other factors that also temper that number. I found the following formula in several blower door manuals (see here, section 6.3.d). The formula introduces two correction factors, one for the efficiency of the heat source and one for everything else.

Annual Heating Cost = (26 x HDD x Fuel Price x CFM50 / N x Seasonal Efficiency) x 0.6

  • 26 is the result of multiplying the heat capacity of air (0.018) x 60 minutes x 24 hours.

  • HDD is the Heating Degree Days (7100).

  • Fuel Price is $/BTU. 1 BTU = 0.000293071 kW. Multiplying times the price of electricity in our area $0.14 kWh gives us 0.0000410299.

  • CFM50 is 245 for 1 ACH50.

  • N is the Energy Climate Factor (14.8).

  • Seasonal Efficiency is the efficiency of the heat source. ASHP range in values from 1-3. Let’s use 2.5. This is one of the correction factors I described earlier.

  • 0.6 is the second correction factor. I found a brief description of what this value represents here.

Using these inputs, we will spend about $30 per heating season just to heat infiltrated air.

If you set Seasonal Efficiency and 0.6 each to 1, then you get roughly the same value I described earlier, $125.

$30 represents the annual cost for air infiltration and it’s less than 6% of the estimated $510 we will spend annually to heat the entire house (space heating only). We can now compare the cost of heating the infiltrated air per heating season for a number of different ACH50 and CFM50 values.

ACH50 / CFM50 0.6 / 137 1 / 245 2 / 469 3 / 704 4 / 939 5 / 1174
Annual cost ($) to heat infiltrated air 17 30 60 90 120 150

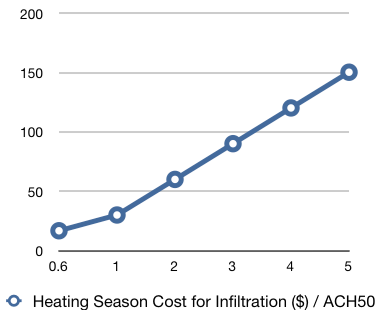
In fact, these numbers should be even less because I’ve included the basement volume in these calculations. The basement is not a heated space but it tempers the temperature in the house.

If we can hit a target of roughly 1 ACH50 we will pay 20% of the cost of an EnergyStar house with 5 ACH50.

You can also compare the different values based on Energy Climate Factor.

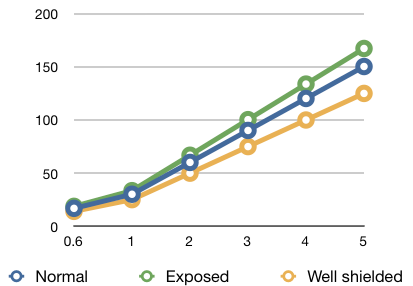
Another way to think about it, our cost is roughly proportional to the amount of air leaked in a blower door test, 6% leakage equals 6% cost of our projected annual heating bill. Saving 6% per year is a lot better than the interest I’m earning on my other investments, and those savings increase as the price of electricity goes up.

Just remember, 6% in this example is based on the expected performance of our house (insulation values, % of window area, orientation, climate, exposure, etc.). A house with lower insulation values, and a warmer climate will find a different % of savings by building a tighter house.